 |
 |
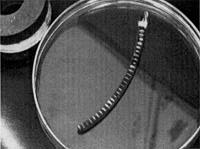
Fig. 1 Shape distortion of a ferrogel, due to non-uniform magnetic
field produced by a permanent magnet:
A) the gel is located 15 cm from the magnet |
|
A |
B |
DIRECT OBSERVATION OF DISCRETE AND REVERSIBLE SHAPE TRANSITION IN MAGNETIC FIELD SENSITIVE POLYMER GELS
Department of Physical Chemistry, Technical University of Budapest,
H-1521 Budapest HUNGARY
Volume phase transition in response to infinitesimal change of external stimuli has been observed in various gels. We report here an abrupt shape transformation occuring in magnetic field sensitive polymer gels, called ferrogels. In a ferrogel finely distributed colloidal particles having superparamagnetic behaviour are incorporated into the swollen network. These particles couple the shape of the gel to the non-uniform external magnetic field. Shape distortion occurs instantaneously and disappears abruptly when the external field is removed. A discontinuous elongation and contraction in response to infinitesimal change in external magnetic field has been observed. The elongation induced by non-uniform magnetic field can be 40% of the initial length. The magneto-elastic properties of ferrogels could be applicable to a variety of fields as a new driving mechanism.
At present there are several adaptive (smart, intelligent) materials that can actuate or alter their properties in response to a changing environment1. Among them mechanical actuators have been the subject of much investigation in recent years2. They undergo a controllable change of shape due to some physical effects and can convert energy (electrical, thermal, chemical) directly to mechanical energy. This can be used to do work against load2-9.
Certain polymer gels represent one class of actuators that have the unique ability to change elastic and swelling properties in a reversible manner. These wet and soft materials offer lifelike capabilities for the future direction of technological development.
Volume phase transition in response to infinitesimal change of external stimuli like pH, temperature, solvent composition,electric field, and light has been observed in various gels14-18 . Their application in devices such as actuators, controlled delivery systems, sensors, separators and artificial muscles has been suggested and are in progress2-8,17-20.
Attempts at developing stimuli-responsive gels for technological purposes are complicated by the fact that structural changes, like shape and swelling degree changes that occur, are kinetically restricted by the collective diffusion of chains and the friction between the polymer network and the swelling agent. This disadvantage often hinders the effort of designing optimal gels for different applications.
In order to accelerate the response of an adaptive gel to stimuli, the use of magnetic field sensitive gels as a new type of actuator has been developed10. Magnetic field sensitive gels, or as we call them "ferrogels", are typical representatives of smart materials.
A ferrogel is a chemically cross-linked polymer network swollen by a ferrofluid11. A ferrofluid, or a magnetic fluid, is a colloidal dispersion of monodomain magnetic particles. Their typical size is about 10 nm and they have superparamagnetic behaviour. In the ferrogel, the finely distributed magnetic particles are located in the swelling liquid and attached to the flexible network chains by adhesive forces. The solid particles of colloidal size are the elementary carriers of a magnetic moment. In the absence of an applied field the moments are randomly oriented, and thus the gel has no net magnetization. As soon as an external field is applied, the magnetic moments tend to align with the field to produce a bulk magnetic moment. With ordinary field strengths, the tendency of the dipole moments to align with the applied field is partially overcome by thermal agitation, such as the molecules of paramagnetic gas. As the strength of field increases, all the particles eventually align their moments along the direction of the field, and as a result, the magnetization saturates. If the field is turned off, the magnetic dipole moments quickly randomize and thus the bulk magnetization is again reduced to zero. In a zero magnetic field a ferrogel presents a mechanical behaviour very close to that of a swollen network filled with non-magnetic colloidal particles.
Preparation of a ferrogel is similar to that of other elastomeric networks. One can precipitate well-dispersed particles in the polymeric material. The "in situ" precipitation can be made before, during and after the cross-linking reaction12. According to another method, the preparation and characterization of colloidal magnetic particles are made separately, and the cross-linking takes place after the polymer solution and the magnetic sol are mixed together10,13. In this paper, chemically cross-linked polyvinyl alcohol hydrogel filled with magnetite particles is reported. At first, magnetite (Fe3O4) sol was prepared from FeCl2 and FeCl3 in aqueous solution. In order to counter-balance the van der Waals attraction and the attractive part of magnetic dipole interactions, colloidal stability has been maintained by a small amount of HClO4 which induced peptization. Then the stabilized magnetite sol, having a concentration of 10 wt% was mixed with polyvinyl alcohol solution. Polyvinyl alcohol (PVA) is a neutral water-swellable polymer which reacts under certain conditions with glutardialdehyde (GDA) resulting in chemical cross-linkages between PVA chains. The cross-linking density can be conveniently varied by the amount of GDA relative to the vinyl alcohol [VA] units of PVA chains. A ferrogel is characterized by the following symbol: sample name / polymer concentration ( given by wt% ) at which the cross-links were introduced / the ratio of vinyl alcohol units to the cross linking molecules / concentration of magnetite particles (given by wt% ). For example, a typical ferrogel, FG/6.3/300/4.25, was prepared at a solution of 6.3 wt% of PVA; the ratio of vinyl alcohol monomer units to the cross linker molecules (GDA) is 300; the magnetite content at preparation is 4.25 wt%.
We have prepared weak PVA gels in order to allow the effect of magnetic interaction on deformation to develop as far as possible. The ratio of [VA] units to [GDA] molecules can be varied between 50 <= [VA]/[GDA] <= 400. A more detailed description of chemical procedure can be found in our previous paper10. Cylindrical samples having a diameter of 1-2 cm and length of 10-20 cm were prepared. These gel tubes were used for the magnetoelastic investigations.
In uniform magnetic field a ferrogel experiences no net force. When it is placed into a spatially non-uniform magnetic field, forces act on the superparamagnetic particles, and the magnetic interactions are enhanced. The stronger field attracts the particles, and due to their small size and strong interactions with molecules of dispersing liquid and polymer chains, they all move together. Because of the cross-linking bridges in the network, changes in molecular conformation can accumulate and lead to macroscopic shape changes and motion. The principle of the ferrogel's shape transformation and motility lies in a unique magneto-elastic behaviour. The magnetic field drives and controls the motion, and the final shape is set by the balance of magnetic and elastic interactions.
 |
 |
Fig. 1 Shape distortion of a ferrogel, due to non-uniform magnetic
field produced by a permanent magnet:
A) the gel is located 15 cm from the magnet |
|
A |
B |
If a ferrogel is placed in a non-uniform magnetic field, as shown in Fig. 1, depending on the field distribution in space, curvature may occur. The gel has its original (straight) shape if the Petri dish containing the ferrogel is far from above the magnet. In this case the magnetic field strength is so small that the magnetic force is too weak to deform the gel. Due to the enhanced magnetic interaction, curvature immediately occurs when the Petri dish is placed on the magnet. The ferrogel can be made to bend and straighten repeatedly many times without damaging the gel. The ability of ferrogels to undergo successive bending and stretching can be used to construct new types of soft actuators as well as worm-like motions20.
Not only curvature, but also stretching and contraction can be realized
by ferrogels in inhomogeneous magnetic field. Let us consider in the Cartesian
coordinate system a non-uniform magnetic field the strength of which varies
only in the direction of z. The measure of this variation
is characterized by the gradient of the magnetic field in space. This field
can be produced by an electromagnet, and the dependence of the field strength
on coordinate z is determined by the steady current flowing through
the electromagnet. Let us suspend vertically (parallel to z)
a cylindrical gel sample. When it is subjected to a nonuniform magnetic
field, a tensile stress develops in the z direction. Due
to this stress - depending on the sign of the field gradient (experimental
arrangement) - elongation or contraction may occur. This is demonstrated
by Fig. 2 where a ferrogel is shown in different types of magnetic
fields produced by two planparallel poles of electromagnets. The position
of the poles of electromagnets can be varied along the direction of z.
We present here four different situations:
A) no magnetic field is applied, B) the axis of magnetic poles is below
the lower end of the gel, C) the axis of magnetic poles is at the top surface
of the ferrogel, D) the axis of the poles is in the middle of the gel along
z.
 |
 |
 |
 |
Fig. 2 Elongation and contraction of a ferrogel in nonuniform
magnetic fields:
A) no magnetic field is applied |
|
A |
B |
C |
D |
In case A) no deformation occurs. In the presence of an applied magnetic field, a field gradient develops parallel to the gel axis and results in elongation in case B) and contraction at arrangements denoted by C) and D).
We have studied the dependence of elongation on the steady current intensity required by the electromagnets to produce an external magnetic field. A cylindrical gel tube was suspended in water to prevent evaporation of swelling liquid and to balance the weight of gels by the buoyancy. The position of the top surface of the ferrogel was fixed at the point zo by a rigid non-magnetic copper thread. This was connected to a force measuring unit in order to discover the force developed in the ferrogel due to a non-uniform magnetic field. The steady current intensity flowing through the electromagnet was varied in order to produce the external magnetic field. The field gradient around the ferrogel was parallel to the axis of the gel tube. The intensity of the current was varied between 0 and 8 A by an electronic device and the voltage was kept constant (20 V). The highest field intensity was 80 mT between the poles of electromagnets, and it disappered within 200 mm.
The elongation of ferrogel was monitored by a CCD camera which allowed us to determine the displacement of the lower-end of the gel with an accuracy of 10-2 mm. Both the elongation and also the force due to magnetic interactions has been measured.
 |
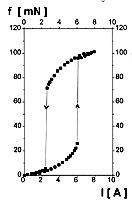 |
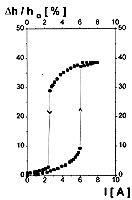
Fig. 3 Non-continuous elongation of a ferrogel FG/5.72/300/4.95
The initial length of the gel, ho is 163 mm, zo = 54.2 mm A) relativ displacement as a function of current |
|
A |
B |
Fig. 3A shows the effect of a magnetic field on the deformation of ferrogel. The relative displacement is plotted against the steady current intensity. It can be seen that the displacement of the lower end of the gel - due to magnetic force - is rather significant. A giant magnetostriction takes place.
In some cases we were able to produce an elongation of 40 % of the initial length by applying a non-uniform magnetic field. It may be seen that at small current intensities the displacement slightly increases. However, at a certain current intensity a comparatively large, abrupt elongation occurs. This non-continuous change in the size of ferrogel appears within an infinitesimal change in the steady current intensity. Further increase in the current intensity results in another small extension. We have found that by decreasing the current a contraction takes place. Similarly to the extension, the measure of the contraction was found to have a non-continuous dependence on current intensity. It is worth mentioning that a significant hysteresis characterizes the extension-contraction processes as seen in Fig. 3. Not only the relative displacement, but also the measured force shows similar dependence characterized by a hysteresis as shown in Fig. 3B.
By variation of the experimental conditions, we have found a cross-over between continuous and discontinuous shape transitions. The initial position in the non-uniform magnetic field seems to play an essential role in the mode of stretching. Fig. 4 shows the dependence of relative displacement as a function of steady current intensity for the same ferrogel in four different experimental arrangements. The position of the top surface, zo, in the magnetic field was varied. It is seen, that with decreasing zo both the meausure of non continuous displacement and the hysteresis keeps on decreasing. At a certain initial position, zo< 21 mm, a continuous shape transformation as a function of current appears.
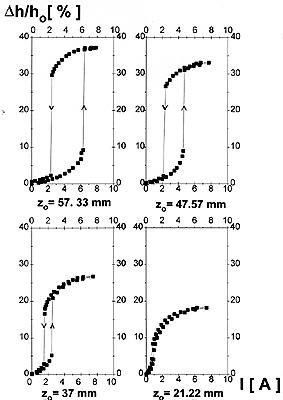 |
Fig. 4 The effect of the initial position on the discontinuous shape transition for the gel FG/5.72/300/2.75. The values of zo are shown in the figures, ho = 164 mm. |
The cross-over between continuous and non-continuous transitions seems to be determined by the position of gel in the non-uniform magnetic field. It is worth mentioning that the discrete shape transition occurred within a time interval of one second, independently from the gel size.
We have shown here that ferrogels undergo quick and reversible shape transformation induced by changes in external magnetic field. Elucidating the mechanism that could account for these phenomena may give rise to a new driving mechanism. According to our findings, ferrogels could be used to make magneto-mechanochemical transducers, sensors, switches, and if the magnetic field is coordinated and controlled by a computer, this magneto-elastic material may be used as an artificial muscle.
ACKNOWLEDGEMENTS
This work was supported by the Hungarian Academy of Sciences under the contract of OTKA T 015754.
References
1. F.Hoke, The Scientist, p.13, Apr.27 (1992)
2. De Rossi, Kawana K., Y. Osada and A. Yamauchi Eds., Polymer Gels Fundamentals and Biomedical Applications, Plenum Press, New York (1991).
3. W.Kuhn, B.Hargitay, A.Katchalsky and H. Eisennberg, Nature, 165, 514 (1950)
4. A.Katchalsky, J. Polym. Sci. 7:393 (1951)
5. Y.Tatara, Bull. JSME 106(17) 451 (1974)
6. M.V.Sussman, Nature, 256, 195 (1975)
7. P.E. De Rossi, P.Chiarelli, G.Buzzigoh, C.Domenici, L.Lazzeri TRans. Am. Soc. Artif. Intern. Organs, Vol. XXXII, 157 (1986)
8. Y.Osada, Advances in Polymer Science 82, 1 (1987)
9. M.Zrínyi, F.Horkay, Journal of Intelligent Material Systems and Structures Vol. 4, 190 (1993)
10. M.Zrínyi, L.Barsi, A.Büki, Polymer Gels and Networks in press
11. R.E.Rosenweig, Ferrohydrodynamics, Cambridge University Press, Cambridge, London, New York, New Rochelle, Melburne, Sydney, (1985)
12. J.E.Mark, British Polymer Journal 17, 144 (1985)
13. W.Haas, M.Zrínyi, H.-G. Kilian, B.Heise, Colloid and Polymer Science, 271, 1024 (1993)
14. K.Dusek and W.Prius, Advances in Polymer Science, 6, 1 (1969)
15. T.Tanaka, Science 218, 467 (1982)
16. T.Tanaka, Phys. Rev. Lett. 40, 820 (1978)
17. K.Dusek (Editor), Advances in Polymer Science, Vol. 109 (1993) Responsive Gels, Volume transitions I.
18. K.Dusek (Editor), Advances in Polymer Science, Vol. 110 (1993) Responsive Gels, Volume transitions II.
19. A.Suzuki, Advances in Polymer Science, Vol. 110, 201 (1993)
20. Y.Osada, H.Okuzaki, H.Hori, Nature, Vol. 355 16.Jan (1992)